Link Search Menu Expand Document
CMI Entrance Exam

MT #4: Limits and derivatives

Timings: 17:00-20:00 Hrs    Date: 10 March 2021

Instructions

  • You are responsible for keeping time. Email all your solutions by 20:00 Hrs IST.
  • Write your answers with a dark pen on white paper.
  • Find an email from me with the subject line ‘Mock test 4: Limits and derivatives’. Send your solutions (images) as replies to this email.
  • Adjust/Reduce the resolution of the camera so that each image is less than 500 KB in size.
  • Total marks: 100 (10x4=40 for Part A + 6x10=60 for Part B)

For students who miss the live test (members only)
Self-administer the mock test and email your solutions before 11 Mar, 23:59 Hrs. Your solutions will be evaluated but marks won’t be counted for official use in the future. Solutions submitted after 11 Mar, 23:59 Hrs will not be evaluated. As per the rules of CMI entrance exam, no calculators or log tables must be used.

Part A: Short-answer type questions

Submission file: Write answers to all the ten questions on a single sheet of paper. Email a picture of your answer sheet. Name the file as PartA.jpg.

For this part, answers must be written without any explanation.

  1. Suppose \(p(x)\) and \(q(x)\) are polynomials such that: \[ p(x) = (x-1)q(x) \] If \( p^{\prime}(1)=1\), what is the value of \( q(1) \)?

  2. Let \(D_1\) denote the set of positive one-digit numbers, \(D_3\) the set of three digit numbers and in general \(D_n\) the set of \(n\)-digit numbers. Consider the following sum for any odd \(n\): \[ S_{n} = \sum_{k_1\in D_1} \frac{1}{k_1} + \sum_{k_3\in D_3} \frac{1}{k_3} + \sum_{k_5\in D_5} \frac{1}{k_5} + \cdots + \sum_{k_n\in D_n} \frac{1}{k_n} \] As \(n\) tends to infinity, to what value does \(S_n\) tend to?

  3. Consider the function \(f: \mathbb{R} \rightarrow \mathbb{R}\) given by \( f(x)=(x-1)|(x-1)(x-2)|\). The function \(f\) is
    1. differentiable at \(x=1\) and \(x=2\).
    2. differentiable at \(x=1\) but not at \(x=2\).
    3. differentiable at \(x=2\) but not at \(x=1\).
    4. neither differentiable at \(x=1\) nor at \(x=2\).
    Pick the right option.

  4. What is the smallest value of \(n\) for which the following limit exists? \[ \lim_{x \rightarrow 0} \frac{\sin^{n}x}{\cos^{4}x(1-\cos x)^{3}} \]

  5. We have a tape that is wound as a spool around axle \(A\). There is an axle \(B\) that winds the tape around itself at constant speed. In other words, a fixed length of tape is transfered in one minute from spool \(A\) to \(B\). Let the instantaneous radius of the spool \(A\) be \(r\). Intially, at \(t=0\) minutes, \(r=R \) cm. At \(t=50\) minutes, \(r=0\) cm. Qualitatively, sketch the value of \(r\) as a function of time.


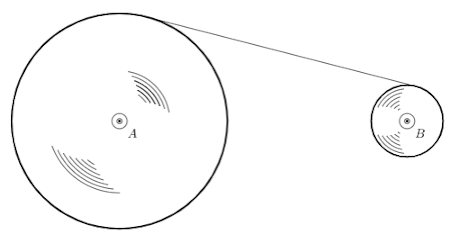
    Sketch the value of \(r\) against time.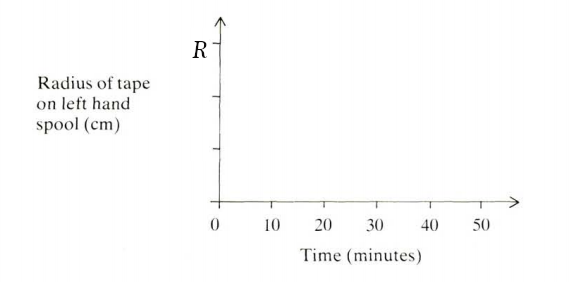

  6. Let \(f_{0}(x)=(\sqrt[3]{e})^{x}\). For \(n\geq 0\), we define \(f_{n+1}(x)=f_{n}^{\prime}(x)\). Compute \( \sum_{i=0}^{\infty} f_{i}(1) \).

  7. Alice and Bob are standing on a playground and Alice is 400m directly East of Bob. Alice starts to walk North at a rate of 3 m/sec, while Bob starts to walk South at the same time at a rate of 7 m/sec. After 30 seconds, at what rate is the distance between Alice and Bob changing?

  8. Suppose \(p(x) = x^3 + x^2 + 2x + k\). Which of the following statements is true?
    1. \( p(x) \) has exactly one real root for any value of \(k\).
    2. For some real number \(k\), \(p(x)\) has more than one real root.

  9. Calculate the value of the infinite series: \[ \sum_{k=1}^{\infty} \frac{1}{2 k^{2}-k} \]

  10. Evaluate: \[ \lim_{n \rightarrow \infty} 4^{n}\left(1-\cos \left(\pi / 2^{n+1}\right) \right) \]

Part B: Subjective questions

Submission files: Each question in this part must be answered on a page of its own. Name the files as B1.jpg, B2.jpg, etc. In case you have multiple files for the same question, say B4, name the corresponding files as B4-1.jpg, B4-2.jpg, etc.

Clearly explain your entire reasoning. No credit will be given without reasoning. Partial solutions may get partial credit.

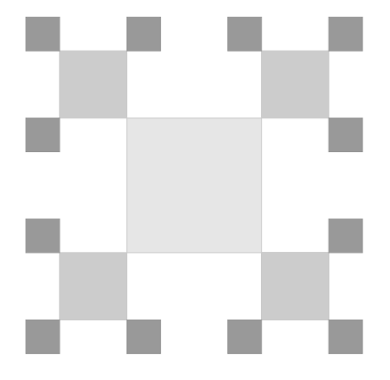
  • B1. Consider an axis-parallel rectangle with its bottom-left corner at the origin and top-right corner on the curve \(y=-\ln 3x\). What is the maximum area that can be attained by any such rectangle?

    B2. A fractal region is constructed as follows. Initially, a unit square is added at \(t=1 s\). At each subsequent time step \(t\), new squares of size \(s_t\) are added to the region as follows.

    For each square \(S\) that was added at time step \(t-1\):

    • Add a square with side length \(s_t = s_{t-1}/2\) to each of the free corners of \(S\).
    The figure below shows the region after the third time step. Squares of the same shade were added at the same time step. As \(t\) tends to infinity, what value does the area of the region approach?

    B3. Let \(x_{0}=1\) and \[ x_{n}=\frac{3+2 x_{n-1}}{3+x_{n-1}} \] for \(n=1,2, \ldots .\)
    (a) Prove that the sequence converges, that is, \( \lim_{n \rightarrow \infty} x_{n} \) exists. [6 marks]
    (b) Find the value of the above limit. [4 marks]

    B4. Find the value of the limit: \[ \lim_{x \rightarrow 0} \frac{1}{x} \ln \left(\frac{e^{x}-1}{x}\right) \]

    B5. A monic polynomial is a polynomial with leading coefficient as 1. Suppose \(p\) is a monic polynomial with real coefficients. Let \( \lim_{x \rightarrow \infty} p^{\prime \prime}(x)=\lim_{x \rightarrow \infty} p\left(\frac{1}{x}\right) \) and \( p(x) \geq p(2) \) for all \( x \in \mathbb{R} \). Find \( p(x) \).

    B6. Let \(p(x)\) be a polynomial with only real roots. Show that for every \(x\): \[ p^{\prime}(x)^2 \geq p(x) p^{\prime\prime}(x) \]