MT #2: Algebra
Timings: 17:00-20:00 Hrs Date: 18 Feb 2021
Part A: Short-answer type questions
Submission file: Write answers to all the ten questions on a single sheet of paper. Email a picture of your answer sheet. Name the file as PartA.jpg.
For this part, answers must be written without any explanation.
- Let \(p_1(x),p_2(x),\ldots,p_m(x)\) be \(m\) distinct polynomials such that:
\[ p_1(x) = p_2(x) = \ldots = p_m(x) \mbox{ if } x<0 \] What is the largest possible value of \(m\)? For example, if it is possible for \(m\) to be 4, then the polynomials might look like this: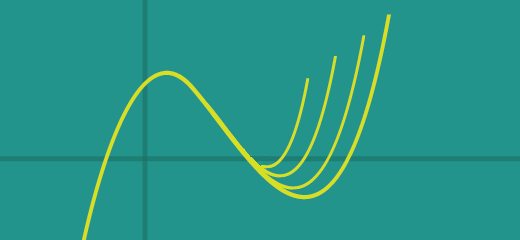
- Find a polynomial \(q(x)\) with integer coefficients with \(\sqrt{3}+i\) as a root. In case there are multiple candidates, pick a polynomial with the least degree.
- (i) Find the remainder when \(f(x)=6x^{16}+4x^{22}+5x^{12}+x^{2}\) is divided by \((x^{2}+1)\). [2 marks]
(ii) Find the remainder when \(x f(x)\) is divided by \((x^{2}+1)\). [2 marks] - Find two quadratic polynomials \( p(x) \) and \( g(x) \) that satisfy the following conditions:
- Both \(p(x)\) and \(g(x)\) have two distinct real roots.
- The sum \( p(x)+g(x) \) has no real root.
- A grazing field has \(10\) kgs of grass. Every Sunday a herd of cows eats \(x\) kgs of grass. Over a week's time the grass grows by \( 10\% \). What is the maximum value of \(x\) that will allow the cows to feed indefinitely without running out of grass?
- Consider the simultaneous equations in variables \(x\) and \(y\), where \(k\) is a constant: \begin{align*} 2x + y &= kx + 4 \\ x + 2y &= ky + 6k \end{align*} For what values of \(k\) does the system not have a solution?
- Notation. \( { }^nC_{k} \) represents the binomial coefficient.
Consider the set of prime numbers less than 100 (listed below). Pick two numbers \(n\) and \(k\) with \(n > k \) from this set such that \( {}^nC_{k} \) is maximized.
- We have numbers \( x_1,\ldots,x_{51} \) such that each \(x_i\) is either \(+1\) or \(-1\). What is the minimum value of following sum? \[ \left\lvert \sum_{ 1\leq i < j \leq 51 } x_ix_j \right\rvert \]Problem source: PRMO 2019.
- Notation. \( [n] \) denotes the set of numbers \( \{1,2,\ldots,n\} \). Assume \(n>100\) for this problem.
A function \(f:[n]\rightarrow \mathbb{R} \) is defined as follows:
\[ f(x) = \begin{cases} 0 &\mbox{if } x = 1 \\ 1 & \mbox{if } x = n \\ \frac{1}{2} (f(x-1) + f(x+1)) & \mbox{if } 1 < x < n \end{cases} \] What is the value of \( f(3) \) in terms of \(n\)? - Let \( f(x) = 37 x^{4}-37 x^{3}-x^{2}+9 x-2 \). Let the four roots of \( f(x) \) be \( r_{1}, r_{2}, r_{3}\) and \( r_{4} \). Find the value of \[ \left(r_{1}+r_{2}+r_{3}\right)\left(r_{1}+r_{2}+r_{4}\right)\left(r_{1}+r_{3}+r_{4}\right)\left(r_{2}+r_{3}+r_{4}\right) \] Problem source: Stanford Math Tournament.
Part B: Subjective questions
Submission files: Each question in this part must be answered on a page of its own. Name the files as B1.jpg, B2.jpg, etc. In case you have multiple files for the same question, say B4, name the corresponding files as B4-1.jpg, B4-2.jpg, etc.
Clearly explain your entire reasoning. No credit will be given without reasoning. Partial solutions may get partial credit.
B1. Prove the following inequality for \( a, b>0 \): \[ \large \sqrt[2021]{a b^{2020}} \leq \frac{ a+2020b }{2021} \]
Problem source: Problem solving strategies. Prob. 4, Inequalities.
B2. Consider the polynomial \(f(x) = a_{n}x^n +a_{n-1}x^{n-1} + \cdots + a_{1}x+a_{0}\), where each coefficient \(a_{i}\) is either \(0\) or \(1\). If \( f(2) = 14\), find the polynomial \(f(x)\).
B3. Compute the smallest value \(p\) such that, for all \(q> p\), the polynomial \( x^3 - 7x^2 +qx +16 = 0 \) has exactly one real root.
Problem source: Stanford Math Tournament.
B4. Let \(f(x)=a_{0} x^{n}+\cdots+a_{n}\) be a non-constant polynomial with real coefficients satisfying \[ f(x) f\left(x^{2}\right)=f\left(x^{3}+x\right) \] for all real numbers \(x\). Show that \(f\) has no real root.
B5. Show that \(p(x)\) does not have any real roots where: \[ p(x)=x^{2 n}-2 x^{2 n-1}+3 x^{2 n-2}-4 x^{2 n-3}+\cdots-2 n x+(2 n+1) \]
Problem source: Problem solving strategies. Prob. 5, Polynomials.
B6. (i) Simplify \[ \sum_{j=0}^{n} \sum_{i=j}^{n}{ }^{n} C_{i}{ }^{i} C_{j} \]
(ii) Calculate the value of the expression (i) when \( n=5 \).
Problem sources for B4 and B6: Madhava Mathematics Competition 2019.